
(9)EF=❝G //(7), Triangle midsegment theorem (8) EF||DG //(7), Triangle midsegment theorem (7) EF is midsegment //(6), definition of midsegment (6) AF=FG //(5), corresponding sides of congruent triangles (2) ∠BAF ≅ ∠CGF // Alternate Interior Angles Theorem
#4. isosceles trapezoid definition geometry how to#
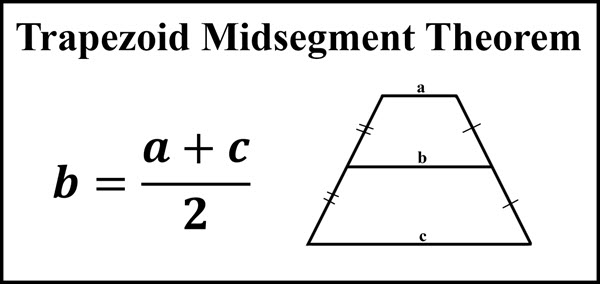
Here's how to prove the trapezoid midsegment theorem: In other words, the length of EF is the arithmetic mean (average) of the lengths of the bases. As such, by the Triangle midsegment theorem, it is parallel to DG and is equal to half of DG.īut DG is DC+ CG, and as ΔABF and ΔGCF are congruent, CG= AB, so EF is equal to half of DC+ AB. From this, we can show that EF is a midsegment of triangle ΔADG.

We can easily show that ΔABF and ΔGCF are congruent, using the Angle-Side-Angle postulate. Let's create such triangles, by drawing a line from the vertex A through the midpoint, F, until it intersects an extension of the base DC at point G: Prove that EF|| DC and that EF=½( AB+ DC) StrategyĪs we are dealing with the midpoints of segments, we will use what we have already proven for triangle midsegments. EF is a line connecting the midpoints of legs AD and BC, AE= ED and BF= FC. There is a similar theorem for trapezoids: a line connecting the midpoints of the two legs of a trapezoid is parallel to the bases, and its length is equal to half the sum of lengths of the bases. The triangle midsegment theorem states that the line connecting the midpoints of two sides of a triangle, called the midsegment, is parallel to the third side, and its length is equal to half the length of the third side. In today's geometry lesson, we will prove the trapezoid midsegment theorem, relying on the previously proven triangle midsegment theorem.


 0 kommentar(er)
0 kommentar(er)
